ISSN 2658–5782
DOI 10.21662
Electronic Scientific Journal


© Институт механики
им. Р.Р. Мавлютова
УФИЦ РАН

Яндекс.Метрика web site traffic statistics

Galiakbarova E.V., Karimova G.R. Vertical polarization surface waves at the boundary of porous media saturated with hydrate and ice. Multiphase Systems. 19 (2024) 4. 152–156 (in Russian).
2024. Vol. 19. Issue 4, Pp. 152–156
URL: http://mfs.uimech.org/mfs2024.4.023,en
DOI: 10.21662/mfs2024.4.023
Vertical polarization surface waves at the boundary of porous media saturated with hydrate and ice
E.V. Galiakbarova∗, G.R. Karimova∗∗
∗Ufa State Petroleum Technological University, Ufa, Russia
∗∗Mavlyutov Institute of Mechanics UFRC RAS, Ufa, Russia

Abstract

This paper considers the process of wave propagation of vertical polarization, there is no horizontal transverse motion, along the interface between two media, where the upper medium is represented by sand saturated with ice, and the lower medium is represented by sand saturated with gas hydrate, at positive temperatures on the Celsius scale and pressures corresponding to the stable existence of gas hydrates according to laboratory data. In the rectangular coordinate system, the axis directions are chosen for the case when the interface plane is z = 0. The harmonic wave propagates at a sufficiently distant distance from the explosion source, which is represented by the sum of longitudinal and transverse waves. To describe the mathematical model we used wave equations, equations for particle displacement and components of stress tensors, which we supplement with boundary conditions. Experimental values of velocities, longitudinal and transverse waves in saturated porous media depending on various parameters of porous media were used for numerical calculations. 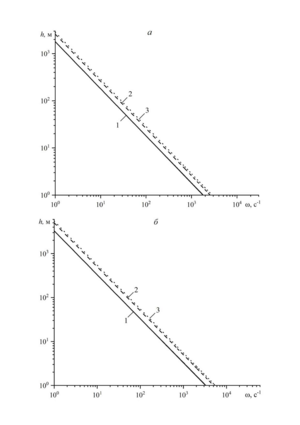The depth of penetration of transverse wave in saturated sand is almost 2 times higher than that of longitudinal wave.

Keywords

surface acoustic waves,
vertical polarization wave,
dispersion equation

Article outline

Surface acoustic waves have received a great deal of attention from researchers, as their applications and fields of study for continuum mechanics are multifaceted. These elastic waves propagate along the free surface of a solid body or a boundary with other media and attenuate at a distance from the boundary. At present, quite a significant contribution to the study of a large number of phenomena is made by the theory of acoustic waves. A considerable number of works are devoted to the physical properties, applications and characteristics of these waves in various media, with various complicating factors. It is known that surface waves come with vertical and horizontal polarization, and the most common special cases include Rayleigh, Lave and Stoneleigh waves. Of greatest interest is the problem of propagation of a surface harmonic wave of vertical polarization along the interface of two media. These waves are studied under different conditions in geophysics, seismology to estimate rock permeability, distribution, reservoir power, etc. With the help of these waves, rock permeability, hydrate formation power can be estimated and the values of vertical polarization wave velocities can be obtained.

In the present work, vertical polarization wave propagation along the interface of two media “porous medium saturated with hydrate — porous medium saturated with ice”. In the rectangular coordinate system, the axis directions are chosen for the case when the interface plane is z = 0. A wave of vertical polarization is considered, i.e. there is no horizontal transverse motion. The harmonic wave propagates to a sufficiently distant distance from the explosion source and is represented by the sum of longitudinal and transverse waves in each half-space. To describe the mathematical model, wave equations, equations for particle displacement and components of stress tensors are used, which are supplemented with boundary conditions. As a result of finding analytical solutions in the form of a harmonic traveling wave for displacements, potentials, longitudinal and transverse wave vectors, a dispersion equation for determining the wave velocity is obtained. Using this equation and experimental values of longitudinal and transverse wave velocities in saturated porous media, it is shown that the penetration depth of the transverse wave into the hydrate-saturated sand medium is about 2 times lower than that of the longitudinal wave.

References

  1. Олинер А.А. Поверхностные акустические волны. Москва: Мир, 1981. 390 с.
    Oliner A.A. Acoustic Surface Waves. Berlin, Heidelberg, New York: Springer-Verlag. 1978. 331 p.
    DOI: 10.1007/3-540-08575-0
  2. Макогон Ю.Ф. Газoгидраты. История изучения и перспективы освоения // Геология и полезные ископаемые мирового океана. 2010. № 2(20). С. 5–21.
    Makogon Y.F. Gas hydrates. History of study and prospects of development // Geology and Mineral Resources of the World Ocean. 2010. № 2 (20). Pp. 5–21. (in Russian)
    EDN: msydrp
  3. Сапарлиев Х.М., Нургельдыев Н. Основные типы сейсмических волн // Новое слово в науке и практике: гипотезы и апробация результатов исследований. 2015. № 18. С. 17–26.
    Saparliev H.M., Nurgeldiev N. Main types of seismic waves // New word in science and practice: hypotheses and approbation of research results. 2015. № 18. Pp. 17–26. (in Russian)
    EDN: ugxolp
  4. Лепендин Л.Ф. Акустика. Москва: Высшая школа, 1978. 448 с.
    Lependin L.F. Acoustics. Moscow: Higher School, 1978. 448 p. (in Russian).
  5. Исакович М.А. Общая акустика. Москва: Наука, 1973. 496 с.
    Isakovich M.A. General acoustics. Moscow: Nauka, 1973. 496 p. (in Russian).
  6. Бреховских Л.М. Волны в слоистых средах. Москва: Наука, 1973. 343 с.
    Brekhovskikh L.M. Waves in layered media. Moscow: Nauka, 1973. 343 p. (in Russian).
  7. Викторов И.А. Звуковые поверхностные волны в твердых телах. Москва: Наука, 1981. 287 с.
    Viktorov I.A. Sound surface waves in solids. Moscow: Nauka, 1981. 287 p. (in Russian).
  8. Гринченко В.Т., Мелешко В.В. Гармонические колебания и волны в упругих телах. К.: Наук. думка, 1981. 284 с.
    Grinchenko V.T., Meleshko V.V. Harmonic vibrations and waves in elastic bodies. K.: Nauk. dumka, 1981. 284 p.
  9. Stoneley R. Elastic Waves at the Surface of Separation of Two Solids // Proceedings of the Royal Society of London. Series A Containing Papers of a Mathematical and Physical Character. 1924. V. 106, No. 738. Pp. 416–428.
    DOI: 10.1098/rspa.1924.0079
  10. Абакумов К.Е., Коновалов Р.С. Распространение акустических волн Стоунли в области границы твердых полупространств при нарушенном акустическом контакте // Известия СПБГЭТУ ЛЭТИ. 2007. № 3. С. 3–7.
    Abbakumov K.E., Konovalov R.S. Distribution of Stoneley acoustic waves near to border of solid half-spaces at the broken acoustic contact // Izvestiya SPBGETU LETI. 2007. No. 3. Pp. 3–7. (in Russian)
    EDN: thjiid
  11. Ilyashenko A.V. Stoneley waves in a vicinity of the Wiechert condition // International Journal of Dynamics and Control. 2021. V. 9, Is. 1. Pp. 30–32.
    DOI: 10.1007/s40435-020-00625-y
  12. Ji J., Milkereit B. Full Waveform Sonic Data From A Fast Formation // CSEQ National Convention. 2004.
    https://old.cseg.ca/assets/files/resources/abstracts/2004/052S0131-Ji_J_Full_Waveform_Sonic_Data.pdf
  13. Губайдуллин А.А., Болдырева О.Ю. Волны в пористой среде со слоем, содержащим газовый гидрат // Прикладная механика и техническая физика. 2020. Т. 61, № 4(362). С. 31–38.
    DOI: 10.15372/PMTF20200404
    Gubaidullin A.A., Boldyreva O.Y. Waves in a porous medium with a gas hydrate containing layer // Journal of Applied Mechanics and Technical Physics. 2020. V. 61, No. 4. P. 525–531.
    DOI: 10.1134/S0021894420040045
  14. Губайдуллин А.А., Болдырева О.Ю., Дудко Д.Н. Скорость и поглощение линейных волн в пористых средах, насыщенных газом и его гидратом // Прикладная механика и техническая физика. 2022. Т. 63, № 4(374). С. 56–63.
    DOI: 10.15372/PMTF20220406
    Gubaidullin A.A., Boldyreva O.Y., Dudko D.N. Velocity and attenuation of linear waves in porous media saturated with gas and its hydrate // Journal of Applied Mechanics and Technical Physics. 2022. V. 63, No. 4. P. 599–605.
    DOI: 10.1134/s002189442204006x
  15. Дучков А.Д., Дучков А.А., Дугаров Г.А., Дробчик А.Н. Скорости ультразвуковых волн в песчаных образцах, содержащих воду, лед или гидраты метана и тетрагидрофурана // Доклады Академии наук. 2018. Т. 478, № 1. С. 94–99.
    DOI: 10.7868/S086956521801019X
    Duchkov A.D., Duchkov A.A., Dugarov G.A., Drobchik A.N. Velocities of Ultrasonic Waves in Sand Samples Containing Water, Ice, or Methane and Tetrahydrofuran Hydrates (Laboratory Measurements) // Doklady Earth Sciences. 2018. V. 478, No. 1. Pp. 74–78.
    DOI: 10.1134/S1028334X18010014