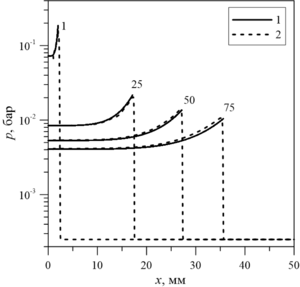
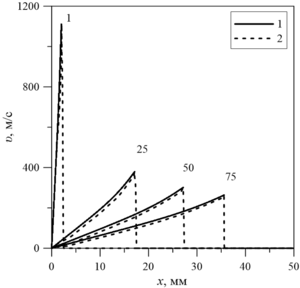
Comparative analysis of solutions of Sedov’s problem of a point explosion in gas for the plane case, obtained by the analytical method and using the open software package of computational fluid dynamics OpenFOAM, is carried out. A brief analysis of methods of dimensionality and similarity theory used for the analytical self-similar solution of point explosion problem in a perfect gas (nitrogen) which determined by the density of uncompressed gas, magnitude of released energy, ratio of specific heat capacities and by the index of geometry of the explosion is given. The system of one-dimensional gas dynamics equations for a perfect gas includes the laws of conservation of mass, momentum, and energy is used. It is assumed that at the initial moment of time there is a point explosion with instantaneous release of energy. Analytical self-similar solutions for the Euler and Lagrangian coordinates, mass velocity, pressure, temperature, and density in the case of plane geometry are given. The numerical simulation of considered process in sonicFoam solver of OpenFOAM package built on the PISO algorithm was performed. For numerical modeling the system of differential equations of gas dynamics is used, including the equations of continuity, Navier-Stokes motion for a compressible medium and conservation of internal energy. Initial and boundary conditions were selected in accordance with the obtained analytical solution using the setFieldsDict, blockMeshDict, and uniformFixedValue utilities. The obtained analytical and numerical solutions have a satisfactory agreement.
point explosion,
self-similar solution,
numerical modeling,
OpenFOAM package,
comparative analysis
Purpose. Comparative analysis of the numerical and self-similar solutions of the Sedov problem on a point explosion in gas.
Methodology. For the numerical solution the sonicFoam solver of the OpenFOAM library is chosen, which is built on the implicit PISO algorithm that calculates pressure with a two-step corrector. In accordance with the mathematical formulation of the problem the computational grid region was formed in a spatial Cartesian coordinate system, the initial and boundary conditions of the problem were set using the setFieldsDict and blockMeshDict utilities. The boundary conditions for pressure, temperature, and velocity as a function of time are generated using the uniformFixedValue function. For numerical modeling the system of differential equations of gas dynamics including the equations of continuity, Navier-Stokes motion for a compressible medium and conservation of internal energy is used.
Findings. The analytical solution to the Sedov’s problem was obtained under the under the following initial conditions: gas-nitrogen with an initial density
ρ1=0.0125 kg/m3, explosion energy
Value. Comparative analysis of analytical and numerical solutions for the problem of a point explosion in the plane case (Sedov's problem) is perfomed. The consistency of the solutions obtained analytically and using the sonicFoam solver of the OpenFOAM package with the initial and boundary conditions constructed on the basis of the investigated self-similar solution is shown. The need for such a comparative analysis is important for evaluating the reliability of the obtained solutions for a wide range of gas-dynamic problems using the considered solver.