Article outline

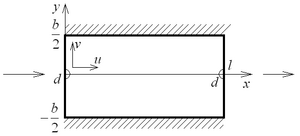
Unstable displacement is an urgent problem in the oil and gas industry and manifests itself when a more viscous fluid is displaced by a less viscous one. For example, the displacement of oil in the formation by water and various agents, the viscosity of which is much less than the viscosity of oil, leading to waterflooding of the formation over time.

The foundations of the current state of research in the theory of filtration of immiscible liquids were laid in the works of Leibenzon L.S. and Musketa M. In these works, the plane-parallel and plane-radial problems of piston displacement of a viscous fluid from a porous medium were first solved. Problems of this kind were further developed in the works of Shchelkachev V.N., Charny I.A., Polubarinova-Kochina P.Ya., Pirverdyan A.M., Barenblatt G.I., Nikolaevsky V.N. and others.

The model for the displacement of immiscible fluids is the Buckley–Leverett model, which is currently the most commonly used in the theory of two-phase fluid filtration. The mathematical model of this process consists of the generalized Darcy's law and the laws of conservation of masses of both immiscible phases. The first of these laws implies the dependence of the phase permeabilities on the volume fraction of each of the immiscible fluids in the pore space. The resulting system of equations is solved taking into account the initial and boundary conditions imposed on the saturation and pressure distribution functions in the phases. The assumption, made in the Buckley-Leverett model, about the absence of a capillary pressure jump at the boundary of the mobile phases, makes it possible to simplify the original system of equations. However, such a simplification leads to the necessity of introducing the saturation discontinuity front first described by Buckley and Leverett.

The Rapoport-Leas model is a more complete immiscible filtration model. The Rapoport-Leas mathematical model contains an additional equation that determines the pressure jump at the phase boundary. Taking into account the influence of capillary forces makes it possible to describe the so-called stabilized zone arising near the interface. The extent of this zone is inversely proportional to the displacement rate. This approach is valid for low displacement rates. Within the framework of the Rapoport-Leas model, a number of important results were obtained regarding the change in oil saturation of reservoirs in the process of their flooding.

When describing two-phase filtration in real media, the issue of the displacement front stability to small disturbances is also important. Experimental studies carried out by Saffman and Taylor and others have shown that the development of perturbations of a flat displacement front in a porous medium when stability is disturbed occurs in the form of indefinitely growing tongues of the displacing fluid.

Experiments on bulk porous media have shown that stability breakdown occurs when the viscosity ratio of interacting liquids exceeds the critical value of 10–15. At the same time, at low displacement rates, perturbations decay even at viscosity ratios greater than the critical one. A mathematical model for the development of languages of the displacing fluid ball was proposed by Barenblatt. It is assumed that this process can be described using the equations of the Buckley-Leverett model, with relative phase permeabilities linearly dependent on the corresponding saturations.

In this paper, we consider a flat channel filled with an incompressible fluid. Over time, another fluid is injected into the channel. The fluids are immiscible. The paper builds a mathematical model of the process of oil displacement by water in a flat channel, which will allow further numerical studies and comparison of the results with the obtained experimental data, using the example of the Hele-Shaw cell. The complexity of describing the mathematical model lies in a wide range of considered spaces from micro-scales to the order of one centimeter.

The resulting system of equations will be solved in further research by difference methods. The obtained numerical results will be compared with experimental ones for further analysis and identification of optimal conditions for increasing the efficiency of oil recovery.

A comprehensive study will allow us to evaluate and analyze the entire process as a whole, as well as to establish flow parameters to improve the efficiency of displacement and increase oil recovery, since in the numerical modeling of the process it is easier to create many independent experiments with the same initial data, in contrast to the experimental study.