ISSN 2658–5782
DOI 10.21662
Electronic Scientific Journal


© Институт механики
им. Р.Р. Мавлютова
УФИЦ РАН

Яндекс.Метрика web site traffic statistics

Privalov L.Yu., Mikhaylenko C.I. The impact of an additional inlet point on the hot outlet side on the vortex tube productivity. Multiphase Systems. 14 (2019) 3. 176–183 (in Russian).
2019. Vol. 14. Issue 3, Pp. 176–183
URL: http://mfs.uimech.org/mfs2019.3.024,en
DOI: 10.21662/mfs2019.3.024
The impact of an additional inlet point on the hot outlet side on the vortex tube productivity
Privalov L.Yu.∗, Mikhaylenko C.I.∗∗
∗Ufa State Aviation Technical University, Ufa, Russia
∗∗Mavlyutov Institute of Mechanics, UFRC of RAS, Ufa, Russia

Abstract

Based on numerical simulation, the production of cold and hot air on a modified countercurrent vortex tube is studied. A feature of the modification under study is an additional air inlet area along the axis of the pipe from the hot outlet side. An additional point of blowing air is designed to redistribute the gas flows at the cold and hot outlets. Computational experiments were performed in the OpenFOAM software package using the sonicFoam solver based on the k−ε turbulence model under the assumption of an ideal gas. 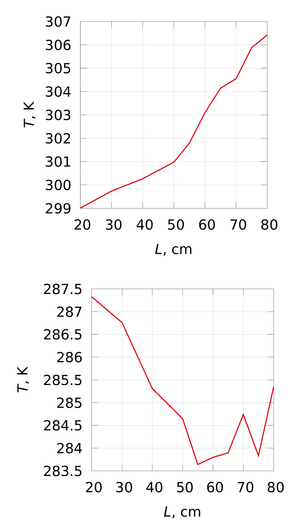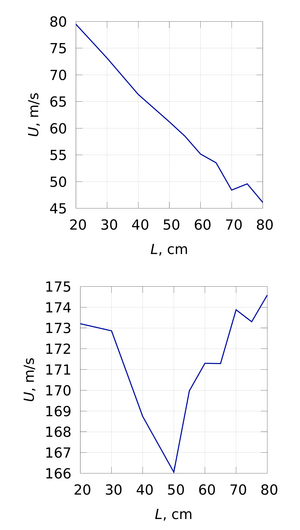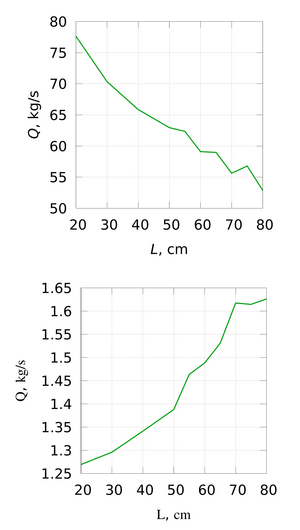The dependence of the flow rate and temperature at the cold and hot outlets for different lengths of the main channel of the vortex tube was studied. For all considered pipe lengths, finite-volume grids were prepared in which the rectangular shape of the cells was preferably observed and their excessive stretching was avoided. To speed up the simulations, MPI technology was used; spatial decomposition of the original mesh was performed by decomposePar utility into equal parts along the pipe. This approach allowed us to reduce the computation time by approximately 3.5 times when running on six processes. The results of parallel modeling were combined with the reconstructPar utility and further processed by a Python program written using the vtk library. Thus, average values of the main physical characteristics by time and space at the cold and hot outlets were obtained. Results are discussed that demonstrate the effect of the vortex tube length on temperature and air flow at the respective outputs. The behavior of its main characteristics, non-standard for a vortex tube, is shown, an assumption is made about the reason for this behavior: the collision of very fast flows makes instability. Preliminary conclusions are made about choosing the effective length of the vortex tube with an additional air inlet channel at which the ratio of air temperature at the hot and cold outlets is the largest.

Keywords

Ranque–Hilsch effect,
vortex tube,
turbulence,
OpenFOAM

Article outline

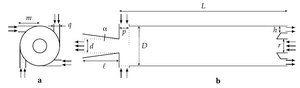
The article considers a vortex tube, supplemented by another air inlet channel. The additional inlet is oriented along the axis of the main channel and is located in its center on the side of the hot diaphragm. The objective of this design is to redistribute the swirling air flows in order to provide the biggest output of cold air.

The mathematical model of the processes under consideration is written on the basis of the equations of continuity, momenta and energy for the case of viscous compressible flow. The system of equations is supplemented by the equation of state of an ideal gas and equations for the kinetic energy of turbulence and the dissipation rate of turbulence. Thus, the turbulent flows inside the vortex tube are described by the k—ε turbulence model.

Computational simulation is performed in the OpenFOAM software using the sonicFoam solver. The choice of a solver is dictated by the fact that transonic flows with shock waves can be realized in the channel of a vortex tube and, especially, in the diaphragm of cold air. In preparing the finite-difference grid, much attention is paid to preserving the orthogonality and uniformity of the sizes of the final volumes. The significant size of the finite difference grid is dictated by the choice of parallel computations using MPI. This approach allows us to accelerate calculations up to 3.5 times with the involvement of 6 processes.

The results show that the additional air inlet channel has a noticeable effect on the redistribution of flows in the vortex tube. However, this effect should be taken into account only for “short” pipes with a main channel length L < 50 cm. The explanation for this effect, apparently, lies in the formation of a soft “piston” directed towards the cold diaphragm in the center of the channel. In general, this is a positive property that can be used to achieve a greater yield of cold air in practice.

References

  1. Hilsch R. The use of the expansion of gases in a centrifugal field as cooling process // Review of Sientific Instruments. 1947. V. 18. Pp. 108–113.
    DOI: 10.1063/1.1740893
  2. Ranque G.J. Experiments on expantion a vortex with simultaneous exhaust of hot air and cold air // J. Phys. Radium. 1933. V. 4. Pp. 112–114 (in French).
  3. Eiamsa-Ard S., Promvonge P. Review of Ranque-Hilsch effects on vortex tubes // Renewable and Sustainable Energy Reviews. 2008. V. 1. Pp. 1822–1842.
    DOI: 10.1016/j.rser.2007.03.006
  4. Subudhi S., Sen M. Review of Ranque–Hilsch vortex tube experiments using air // Renewable and Sustainable Energy Reviews. 2015. V. 52. Pp. 172–178.
    DOI: 10.1016/j.rser.2015.07.103
  5. Thakare H.R., Monde A., Parekh A.D. Experimental, computational and optimization studies of temperature separation and flow physics of vortex tube: A review // Renewable and Sustainable Energy Reviews. 2015. V. 52. Pp. 1043–1071.
    DOI: 10.1016/j.rser.2015.07.198
  6. Borisoglebskiy I.K., Metusova M.V., Mikhaylenko C.I. The dependence of the Ranque–Hilsch effect on the cold outlet geometry // Multiphase Systems. 2018. V. 13, No. 3. Pp. 52–58 (in Russian).
    DOI: 10.21662/mfs2018.3.008
  7. Xue Y., Arjomandi M., Kelso R. A critical review of temperature separation in a vortex tube // Experimental Thermal and Fluid Science. 2010. V. 34, No. 8. Pp. 1367–1374.
    DOI: 10.1016/j.expthermflusci.2010.06.010
  8. Majidi D., Alighardashi H., Farhadi F. Best vortex tube cascade for highest thermal separation // International Journal of Refrigeration. 2018. V. 85. Pp. 282–291.
    DOI: 10.1016/j.ijrefrig.2017.10.006
  9. Moraveji A., Toghraie D. Computational fluid dynamics simulation of heat transfer and fluid flow characteristics in a vortex tube by considering the various parameters // International Journal of Heat and Mass Transfer. 2017. V. 113. Pp. 432–443.
    DOI: 10.1016/j.ijheatmasstransfer.2017.05.095
  10. Mikhaylenko C.I. Dependence of the temperature distribution in the vortex tube on the geometry of the swirler // Proceedings of the Mavlyutov Institute of Mechanics. 2017. V. 12, No. 2. Pp. 174–179 (in Russian).
    DOI: 10.21662/uim2017.2.026
  11. Mikhaylenko C.I. Simulation of the vortex tube: design of a hexagonal mesh for computational experiments in OpenFOAM // Proceedings of the Mavlyutov Institute of Mechanics. 2016. V. 11, No. 1. Pp. 112–118 (in Russian).
    DOI: 10.21662/uim2016.1.017
  12. Mikhaylenko C.I. Vortex tube modelling: outlet parameter dependencies of cold air production // Journal of Physics: Conference Series. 2019. V. 1158, No. 3. 032032.
    DOI: 10.1088/1742-6596/1158/3/032032
  13. Gutsol A.F. The Ranque effect // Physics-Uspekhi. 1997. V. 40. Pp. 639–658.
    DOI: 10.1070/PU1997v040n06ABEH000248
  14. Khait A., Noskov A., Alekhin V., Bianco V. Analysis of the local entropy generation in a double-circuit vortex tube // Applied Thermal Engineering. 2018. V. 130. Pp. 1391–1403.
    DOI: 10.1016/j.applthermaleng.2017.11.136
  15. Rafiee S., Sadeghiazad M. Experimental and 3D CFD investigation on heat transfer and energy separation inside a counter flow vortex tube using different shapes of hot control valves // Applied Thermal Engineering. 2017. V. 110. Pp. 648–664.
    DOI: 10.1016/j.applthermaleng.2016.08.166
  16. Liu J., Chen S., Gan M., Chen Q. Heat transfer and flow resistance characteristics inside an innovative vortex enhanced tube // Applied Thermal Engineering. 2018. V. 144. Pp. 702–710.
    DOI: 10.1016/j.applthermaleng.2018.04.082
  17. Launder B.E., Spalding D.B. The Numerical Computation of Turbulent Flows // Computer Methods in Applied Mechanics and Engineering. 1974. V. 3, No. 2. Pp. 269–289.
    DOI: 10.1016/0045-7825(74)90029-2
  18. Adiullin B.R., Mikhaylenko C.I. The influence of the vortex tube channel length on the separation of air by its temperature. Multiphase Systems. 14 (2019) 1. 36–43 (in Russian).
    DOI: 10.21662/mfs2019.1.005
  19. Adiullin B.R., Mikhaylenko C.I. Influence of the channel length of a vortex tube on the air temperature separation // Journal of Physics: Conference Series. 2019. V. 1268. 012001.
    DOI: 10.1088/1742-6596/1268/1/012001