ISSN 2658–5782
DOI 10.21662
Electronic Scientific Journal





© Институт механики
им. Р.Р. Мавлютова
УФИЦ РАН

Яндекс.Метрика web site traffic statistics

Utyashev I.M., Akhtyamov A.M. Determination of local inhomogeneity of the medium from the natural frequencies of string oscillations. Multiphase Systems. 13 (2018) 4. 99–106.
2018. Vol. 13. Issue 4, Pp. 99–106
URL: http://mfs.uimech.org/mfs2018.4.014,en
DOI: 10.21662/mfs2018.4.014
Determination of local inhomogeneity of the medium from the natural frequencies of string oscillations
Utyashev I.M.∗, Akhtyamov A.M.∗,∗∗
∗Mavlutov Institute of Mechanics, UFRC RAS, Ufa
∗∗Bashkir State University, Ufa

Abstract

The paper considers the problem of determining the local inhomogeneity of the medium from the natural frequencies of string oscillation. The inhomogeneity is modeled in three sections: in the first and third sections medium is homogeneous, and on average section the elastic characteristics are modeled by a quadratic function. This model is implemented using the conjugation conditions at boundary between media. It is shown that to identify the center of an inhomogeneity and determine its size, two natural frequencies are enough, and in the case of rigid fixing of both ends of the string, the solution of the problem is dual. The problem is solved by expanding the fundamental system of solutions into a power series in the variables x and λ. 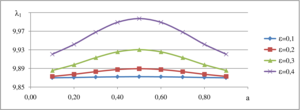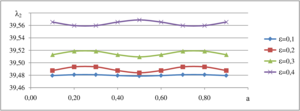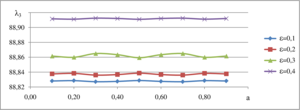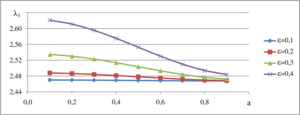The estimates of the error of the method are given.

Keywords

eigenvalues,
Sturm-Liouville problem inverse problem,
potential,
string,
conjugation conditions,
characteristic determinant

Article outline

Purpose: Determination of local heterogeneity of the medium from the natural frequencies of string oscillations.

Methods: The problem of oscillation of a string rigidly fixed at the ends in a medium with local inhomogeneity is reduced to the Sturm-Liouville problem. The inhomogeneity is modeled in three sections: in the first and third sections medium is homogeneous, and on average section the elastic characteristics are modeled by a quadratic function. Using the conjugation conditions at the boundary of the media, a characteristic equation is obtained. The method of solution is based on expanding the fundamental system of solutions into a power series in the variables x and λ. The estimation of the error of the result is made using the noise of the input data, where the noise is given as a function of a random variable with a uniform distribution law. The methods of spectral theory and methods of the theory of inverse problems are also used in the work.

In a study was determined:

1. To determine the parameters of the local inhomogeneity of the medium described by a quadratic function, two eigenfrequencies are sufficient.

2. In the case when the boundary conditions are symmetric, then there are two solutions to the problem.

3. To find a solution to the inverse problem, it is required that the error in the input data should be no more than five decimal places.

References

  1. Ambarzumijan V.A. Uber eine Frage der Eigenwerttheorie // Zeitshrift fur Physik. 1929. Vol. 53, No 9–10. Pp. 690–695.
    (DOI: 10.1007/BF01330827)
  2. Borg G. Eine Umkehrung der Sturm Liouvilleschen Eigenwertanfgabe // Acta Math. 1946. Vol. 78, No 1. Pp. 1–96.
    (DOI: 10.1007/BF0242160)
  3. Levinson N. The inverse Sturm–Liouville problem // Math. Tidsskr. B. 1949. Pp. 25–30.
    (https://www.jstor.org/stable/24527827)
  4. Левитан Б.М. Об определении дифференциального уравнения Штурма–Лиувилля по двум спектрам // Изв. АН СССР, сер. матем. 1964. Т. 28, №1. C. 63–78.
    (http://mi.mathnet.ru/izv3064)
  5. Левитан Б.М., Гасымов М.Г. Определение дифференциального уравнения по двум спектрам // УМН. 1964. Т. 19, №2(116). C. 3–63.
    (http://mi.mathnet.ru/umn6187)
  6. Марченко В.А. Операторы Штурма–Лиувилля и их приложения. Киев: Наукова думка, 1977. 329 c.
  7. Левитан Б.М. Обратные задачи Штурма–Лиувилля и их приложения. М.: Наука, 1984. 240 c.
  8. Шкаликов А.А., Савчук А.М. Обратные задачи для оператора Штурма–Лиувилля с потенциалами из пространств Соболева. Равномерная устойчивость // Функц. анализ и его прил. 2010. Т. 44, №4. C. 34–53.
    (DOI: 10.4213/faa3022)
  9. Юрко В.А. Введение в теорию обратных спектральных задач. М: Физматлит, 2007. 384 c.
  10. Гусейнов И.М., Набиев И.М. Обратная спектральная задача для пучков дифференциальных операторов // Математический сборник. 2007. Т. 198, №11. С. 47–66.
    (DOI: 10.4213/sm1491)
  11. Набиев И.М. Обратная квазипериодическая задача для оператора диффузии // ДАН. 2007. Т. 415, №2. С. 168–170.
    (https://elibrary.ru/item.asp?id=9533626)
  12. Садовничий В.А. Единственность решения обратной задачи в случае уравнения второго порядка с нераспадающимися условиями, регуляризованные суммы части собственных чисел. Факторизация характеристического определителя // ДАН СССР. 1972. Т. 206, №2. С. 293–296.
    (http://mi.mathnet.ru/dan37122)
  13. Плаксина О.А. Обратные задачи спектрального анализа для операторов Штурма–Лиувилля с неразделенными граничными условиями // Матем. сб. 1986. Т. 131(173), №1(9). С. 3–26.
    (http://mi.mathnet.ru/msb1897)
  14. Плаксина О.А. Обратные задачи спектрального анализа для операторов Штурма–Лиувилля с неразделенными граничными условиями. II // Матем. сб. 1988. Т. 136(178), №1(5). С. 140–159.
    (http://mi.mathnet.ru/msb1733)
  15. Гасымов М.Г., Гусейнов И.М., Набиев И.М. Обратная задача для оператора Штурма–Лиувилля с неразделенными самосопряженными граничными условиями // Сибирский математический журнал, 1991. Т. 31, No 6, C. 46–54.
  16. Коротяев Е.Л., Челкак Д.С. Обратная задача Штурма–Лиувилля со смешанными краевыми условиями // Алгебра и анализ. 2009. Т. 21, No 5. C. 114–137.
    (http://mi.mathnet.ru/aa1155)
  17. Mamedov Kh.R., Cetinkaya F. Inverse problem for a class of Sturm-Liouville operator with spectral parameter in boundary condition // Bound. Value Probl. 2013. Article ID 183. 16 p., electronic only.
    (DOI: 10.1186/1687-2770-2013-183)
  18. Panakhov E.S., Koyunbakan H., Unal Ic. Reconstruction formula for the potential function of Sturm–Liouville problem with eigenparameter boundary condition // Inverse Problems in Science and Engineering. 2010. Vol. 18, No 1. P. 173–180.
    (DOI: 10.1080/17415970903234976)
  19. Ахтямов А.М. К единственности решения одной обратной спектральной задачи // Дифференциальные уравнения. 2003. Т. 39. №8. C. 1011–1015.
    (http://mi.mathnet.ru/de10883)
  20. Утяшев И.М., Ахтямов А.М. Идентификация краевых условий струны по собственным частотам колебаний // Труды Института механики им. Р.Р. Мавлютова Уфимского научного центра РАН. 2016. Т. 11. №1. С. 38–52.
    (DOI: 10.21662/uim2016.1.007)
  21. Ахтямов А.М., Утяшев И.М. Восстановление линейного потенциала в задаче Штурма–Лиувилля // Труды Института механики им. Р.Р. Мавлютова Уфимского научного центра РАН. 2017. Т. 12, № 2. С. 152–156.
    (DOI: 10.21662/uim2017.2.022)
  22. Ахтямов А.М., Утяшев И.М. Восстановление полиномиального потенциала в задаче Штурма–Лиувилля // Журнал Средневолжского математического общества. 2018. Т.20, №2. С. 148–158.
    (DOI: 10.15507/2079-6900.20.201802.148-158)