Islamov A.I., Nabiullina K.R. Modeling of thermoconvection in a microtube under point heating:
computational grid preparation and choice of method. Multiphase Systems. 19 (2024) 2. 64–72 (in Russian).
Modeling of thermoconvection in a microtube under point heating: computational grid preparation and choice of method
A.I. Islamov, K.R. Nabiullina
Ufa University of Science and Technology, Ufa, Russia
Abstract
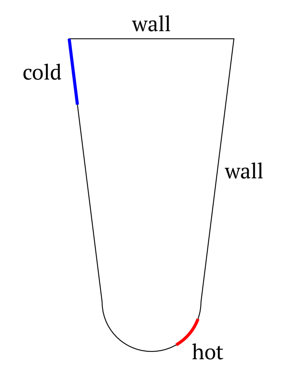
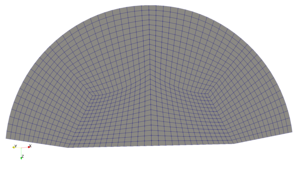
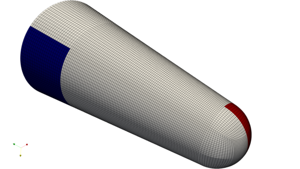
This paper presents the results of the preparatory stage of modeling the process of thermal convection in microtubes. The finite-volume
mesh for a cone-shaped microtube for use within the OpenFOAM software package has been constructed. The obtained mesh was
analyzed and tested. An important stage of this work is the analysis of built-in solvers from the OpenFOAM software package, as well
as the selection of the optimal solver for solving this particular problem. The advantages and disadvantages of two standard solvers —
buoyantBoussinesqPimpleFoam and buoyantPimpleFoam - are considered. It is shown that buoyantBoussinesqPimpleFoam is sufficient
for the considered problem, provided that the program is executed using computations based on OpenMPI libraries, which allows to
significantly reduce the time of computational experiments. Thus, according to the results of the conducted research, a three-dimensional
model of the microtube was prepared, its testing was carried out and the built-in solver from the OpenFOAM software package was
selected. The use of the obtained results can have a significant impact on further research in the field of temperature convection in
microtubes, and consequently in the processes of optimization of PCR.
KeywordsPCR,
polymerase chain reaction,
OpenFOAM,
OpenMPI,
thermal convection,
microfluidics
Article outline
Modern developments in thermoconvection represent a promising approach to accelerating the PCR process. This method is based on active stirring of the reaction mixture, which provides more uniform temperature distribution and improves contacts between reagents. This significantly reduces the heating and cooling time of samples, which is critical for the denaturation, annelination and elongation steps. The use of thermoconvection systems can potentially reduce PCR time to 5-10 minutes, which opens new horizons for the use of this technology in clinical and research practice.
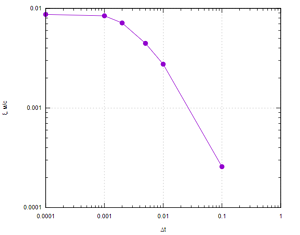
The main objective of this study was to construct a finite-volume mesh for modeling viscous fluid dynamics in microtubes and to select an appropriate modeling tool. To achieve this goal, the following tasks were set: to build a finite-volume model of a microtube to describe thermal convection using OpenFoam software; to analyze the mathematical model; to perform a comparative analysis of two built-in solvers: buoyantBoussinesqPimpleFoam and buoyantPimpleFoam; to run the solver for the obtained mesh; to evaluate the convergence of the built model and its applicability in the studied range of parameters.
According to the results of the analysis of the used mathematical model, the following was revealed: convection is present and the obtained model can be considered adequate, as well as in this model the laminar mode of fluid motion is observed. According to the results of construction and analysis of the grid model, the results were obtained, based on which we can conclude that the topology of the model guarantees the correctness of the structure and boundary conditions, therefore the model can be considered adequate and applicable for use in further studies.
An important stage of this work is the analysis of built-in solvers from the OpenFoam software package, as well as the selection of the optimal solver for solving this particular problem. The advantages and disadvantages of two standard solvers, buoyantBoussinesqPimpleFoam and buoyantPimpleFoam, are considered in this paper. The buoyantBoussinesqPimpleFoam is selected as the optimal solver. The optimal choice of the buoyantBoussinesqPimpleFoam solver is based on its ability to account not only for convective fluxes but also for temperature gradients, which is particularly important for processes involving thermal changes in the reaction mixture. This solver uses the Boussinesq approach, providing a more accurate modeling of convective processes in viscous fluids and allowing high convergence rates.
Thus, this article presents the results of the preparatory stage of work in the study of the promising field of thermoconvection in the context of accelerating the polymerase chain reaction (PCR) process. The use of active stirring of the reaction mixture allows to significantly reduce the time of heating and cooling of samples, which opens new opportunities for the application of this technology in clinical and scientific spheres. The results of this work open new perspectives for the application of thermoconvection in the acceleration of reaction processes and emphasize the importance of choosing the appropriate modeling tool to achieve accuracy and efficiency of research in this field.
References
- Петров А.И. Исследование и практическая реализация программно-аппаратных средств проведения полимеразной цепной реакции с
наблюдением в реальном времени. Санкт-Петербург, 2016. 112 с.
Petrov A.I. [Research and practical implementation of software and hardware for conducting polymerase chain reaction with real-time monitoring]
Issledovanie i prakticheskaja realizacija progrmmno-apparatnyh srdstv provedenia polimerznoj cepnoj reakcii s nabljudeniem v real’nom vremeni.
St. Petersburg. 2016. P. 112.
- Kleppe K.E., Ohtsuka R., Kleppe I. Molineux and Khorana, Studies on polynucleotides. XCVI. Repair replications of short synthetic DNA’s as catalyzed
by DNA polymerases // J. Mol. Biol. 1971. V. 56. Pp. 341–346.
DOI: 10.1016/0022-2836(71)90469-4
- Singhl J., Birbian N., Sinha S., Goswami A. A critical review on PCR, its types and applications // Int. J. Adv. Res. Biol.Sci. 2014. V. 1, No. 7. Pp. 65–80.
https://ijarbs.com/pdfcopy/oct2014/ijarbs11.pdf
- Saiki R.K., Scharf S., Faloona F., Mullis K.B., Horn G.T., Erlich H.A., Arnheim N. Enzymatic amplification of globin genomic sequences and restriction
site analysis for diagnosis of Sickle Cell Anemia // Science. 1985. V. 230. Pp. 1350–1354.
DOI: 10.1126/science.299998
- Simmonds P., Balfe P., Peutherer J.F., Ludlam C.A., Bishop J.O., Brown A.J. Human immunodeficiency virus-infected individuals contain provirus in small
numbers of peripheral mononuclear cells and at low copy numbers // J. Virol. 1990. No. 64. Pp. 864–872.
DOI: 10.1128/jvi.64.2.864-872.1990
- Sykes P.J., Neoh S.H., Brisco M.J., Hughes E., Condon J., Morley A.A. Quantitation of targets for PCR by use of limiting dilution // Biotechniques. 1992.
No. 13. Pp. 444–449.
https://www.academia.edu/download/39689043/Quantitation_of_targets_for_PCR_by_use_o20151104-16311-gtud2z.pdf
- Morley A.A. Digital PCR: A brief history // Biomolecular Detection and Quantification. 2014. No. 1. Pp. 1–2.
DOI: 10.1016/j.bdq.2014.06.001
- Higuchi R., Dollinger G., Walsh P.S., Griffith R. Simultaneous Amplification and Detection of Specific DNA Sequences // Nat. Biotechnol. 1992. No. 10.
Pp. 413–417.
https://www.nature.com/articles/nbt0492-413
- Patent No. US 6,586,233B2, Int. Cl. C12M 1/36. Convectively driven PCR thermal-cycling : No. 09/802,549 : register 09.03.2001 : published 01.07.2003 /
Bennett W.J., Richards J.B., Milanovich F.P.
URL: https://patents.google.com/patent/US6586233B2/en
(accessed: 19.08.2024).
- Patent No. US 5,994,056 A, Int. Cl. C12P 1/48; C12P 1/68; C12N 15/10. Homogeneous methods for nucleic acid amplification and detection : No.
07/695,201 : register 02.05.1991 : published 30.11.1999 / Higuchi R.G.
URL: https://patents.google.com/patent/US5994056A/en
(accessed: 19.08.2024).
- Патент № 2413770 Российская Федерация, МПК C12P 19/34 (2006.01), C12Q 1/68 (2006.01). Способ проведения полимеразной цепной реакции
с помощью конвекции : № 2007121893/10: заявл. 14.06.2007 : опубл. 20.12.2008 / Чемерис Д.А., Чемерис А.В., Магданов Э.Г., Гарафутдинов Р.Р.,
Вахитов В.А., Урманчеев С.Ф., Лебедев Ю.А.
Patent No. RU 2413770 C2, Int. Cl. МПК C12P 19/34 (2006.01), C12Q 1/68 (2006.01). Method of carrying out polymerase chain reaction by means of
convection : No. 2007121893/10: register 14.06.2007 : published 20.12.2008 / Chemeris D.A., Chemeris A.V., Magdanov E.G., Garafutdinov R.R., Vakhitov
V.A., Urmancheev S.F., Lebedev Ju.A.
URL: https://elibrary.ru/download/elibrary_37744165_76189553.pdf
(accessed: 19.08.2024).
- Wu J., Cao W., Wen W. et al. Polydimethylsiloxane microfluidic chip with integrated microheater and thermal sensor // Biomicrofluidics. 2009. V. 3.
P. 012005.
DOI: 10.1063/1.3058587
- Patent No. US 2006/0216725 A1, Int. Cl. CI2O I/68 (2006.01); CI2P 19/34 (2006.01); CI2M I/34 (2006.01). Polymer chain reaction apparatus using
Marangoni covection and polymer chain reaction method using the same : No. 11/219,182 : register 02.09.2005 : published 28.09.2006 / Lee Y-S., Kuk
K., Oh Y-S., Shih S-H., Kim M-S.
URL: https://patents.google.com/patent/US20060216725A1/en
(accessed: 19.08.2024).
- Matsubara Y., Kerman K., Kobayashi M. et al. Microchamber array based DNA quantification and specific sequence detection from a single copy via
PCR in nanoliter volumes // Biosens. Bioelectron. 2005. V. 20. Pp. 1482–1490.
DOI: 10.1016/j.bios.2004.07.002
- Симахина М.А. Условия возникновения и методика расчета параметров конвекции в атмосфере: Автореф. дис. ... канд. физ.-мат.наук: 25.00.30.
Ставрополь, 2010. 21 с.
Simakhina M.A. [Conditions of occurrence and methods of calculating parameters of convection in the atmosphere] Uslovija vozniknovenija i
metodika rascheta perametrov konvekcii v atmosfere. Ph.D theses (Phys. & math). Stavropol. 2010. 21 p.
- Гарифуллин Ф.А. Возникновение конвекции в горизонтальных слоях жидкости // Соросовский образовательный журнал. 2000. Т. 6, № 8. С. 108–114.
Garifullin F.A. [The occurrence of convection in horizontal layers of liquid] Vozniknovenie konvekcii v gorizontal’nyh slojah zhidkosti. Soros
educational journal. 2000. V. 6(8). Pp. 108–114.
- Hopwood A.J., Hurth C., Yang J., Cai Z., Moran N., LeeмEdghill J.G., Nordquist A., Lenigk R., Estes M.D., Haley J.P., McAlister C.R., Chen X., Brooks C.,
Smith S., Elliott K., Koumi P., Zenhausern F., Tully G. Integrated Microfluidic System for Rapid Forensic DNA Analysis: Sample Collection to DNA
Profile // Analyt. Chem. 2010. V. 82, No. 16. Pp. 6991–6999.
DOI: 10.1021/ac101355r
- Гетлинг А.В. Формирование пространственных структур конвекции Рэлея–Бенара // Успехи физ. наук. 1991. Т. 161, № 9. С. 1–80.
DOI: 10.3367/UFNr.0161.199109a.0001
Getling A.V. Formation of spatial structures in Rayleigh—B?nard convection. Sov. Phys. Usp. 1991. 34 (9). Pp. 737–776
DOI: 10.1070/PU1991v034n09ABEH002470
- Антонова О.С., Рудницкая Г.Е., Тупик А.Н., Буляница А.Л., Евстрапов А.А., Курочкин В.Е. Полимеразная цепная реакция: приборная и методическая
реализация. Обзор аналитических характеристик // Научное приборостроение. 2011. Т. 21, № 4. С. 5–21.
Antonova O.S., Rudnitskaya G.E., Tupik A.N., Bulyanitsa A.L., Evstrapov A.A., Kurochkin V.E. Polymerase chain reaction: devices and methods. A
systematic review of literature. Nauchnoe Priborostroenie (Scientific Instrumentation). 2011. V. 21 (4). Pp. 5–21.
EDN: oipjzn
- Тупик А.Н., Курочкин В.Е., Евстрапов А.А. и др. Стеклянные чипы для ПЦР в геле // XIX Менделеевский съезд по общей и прикладной химии
(Волгоград, 25–30 сент. 2011). Сб. материалов. 2011. Т. 4. С. 294.
Tupik A.N., Kurochkin V.E., Evstrapov A.A. et.al. [Glass chips for PCR in gel] Stekljannye chipy dlja PCR v gele. Proc. of XIX Mendeleev simposium of
common and applied chemistry. 2011. V. 4. Pp. 294.
- Золотов Ю.А., Курочкина В.Е. Микрофлюидные системы для химического анализа. Москва: ФИЗМАТЛИТ, 2011. 528 с.
Zolotov Yu.A., Kurochkina V.E. [Microfluidic systems for chemical analysis] Mikrofljuidnye sistemy dlja himicheskogo analiza. Moscow. 2011. 528 p.
- Евстрапов А.А., Буляница А.Л., Рудницкая Г.Е., Лукашенко Т.А., Тупик А.Н., Цымбалов А.И., Есикова Н. А., Посмитная Я.С. Оценка результатов
полимеразной цепной реакции в реальном времени кДНК онкомаркеров СК-19 методом электрофореза на микрофлюидном чипе // Научное
приборостроение. 2012. Т. 22, № 4. С. 77–85.
Evstrapov A.A., Byljanica A.L., Rudnitskaya G.E., et.al. The estimate of results of the real-time polymerase chain reaction of tumor markers cytokeratin-
19 (CK-19) cdna by electrophoresis method in microfluidic chip . Nauchnoe Priborostroenie (Scientific Instrumentation). 2012. V. 22 (4). Pp. 77–85.
EDN: pgghtv
- Boussinesq J. Theorie de l’coulement tourbillonnant et tumultueux des liquides dans les lits rectilignes a grande section. Gauthier-Villars. 1897. 64 p.
- OPENFOAM® (дата обращения 10.04.2024).
https://openfoam.org/
- Mikhaylenko C.I. Building a finite-difference mesh and selecting a turbulence model for numerical simulations of a vortex tube in OpenFOAM
software // Journal of Physics: Conference Series. 2020. V. 1677. 012021.
DOI: 10.1088/1742-6596/1677/1/012021
- Нуриев А.Н., Зайцева О.Н., Камалутдинов А.М., Жучкова О.С. Введение в компьютерное моделирование гидродинамических процессов в
программном комплексе OpenFOAM: учебное пособие. Казань: Издательство Казанского университета, 2022. 84 с.
Nuriev A.N., Zaitseva O.N., Kamalutdinov A.M., Zhuchkova O.S. [Introduction to computer modeling of hydrodynamic processes in the OpenFOAM
software package] Vvedenie v kompjuternoe modelirovanie gidrodinamicheskih processov d programmnom komplekse OpenFOAM. Kazan. 2022. 84 p.
- Кулешов В.С., Моисеев К.В., Урманчеев С.Ф. Изолированные режимы течений при конвекции аномально термовязкой жидкости в плоской
ячейке // Прикладная математика и механика. 2019. № 3. С. 484–494.
DOI: 10.1134/S0032823519030093
Kuleshov V.S., Moiseev K.V., Urmancheev S.F. Isolated Convection Modes for the Anomalous Thermoviscous Liquid in a Plane Cell // Fluid Dynamics.
2019. V. 54, No. 7. Pp. 983–990.
DOI: 10.1134/S0015462819070097
- Кулешов В.С., Моисеев К.В. Конвекция термовязкой жидкости в ячейке, подогреваемой сбоку // Многофазные системы. 2019. T. 14, № 1. С. 67–72.
Kuleshov V.S., Moiseev K.V. Convection of thermoviscouse fluid in a cell heated from the side. Multiphase Systems. 14 (2019) 1. 67–72.
DOI: 10.21662/mfs2019.1.010
- Кулешов В.С. Стационарные режимы конвекции жидкости с гауссовской зависимостью вязкости от температуры // Труды Института механики
им. Р.Р. Мавлютова Уфимского научного центра РАН. 2016. T. 11, № 2. С. 218–225.
Kuleshov V.S. Stationary fluid convection modes with a Gaussian viscosity dependence of temterature Proceedings of the Mavlyutov Institute of
Mechanics. 2016. 11(2). 218–225.
DOI: 10.21662/uim2016.2.032