Delev V.A.
Dynamics of dislocations in the domain structure of the nematic liquid crystal. Multiphase Systems. 15 (2020) 3–4. 201–207 (in Russian).
Dynamics of dislocations in the domain structure of the nematic liquid crystal
Delev V.A.
Institute of Molecule and Crystal Physics UFRC RAS, Ufa
Abstract
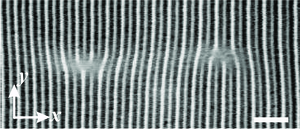
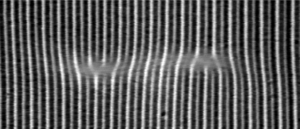
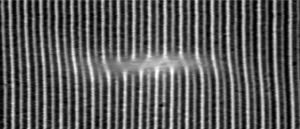
Dynamics and interaction of classical dislocations in the domain structure of π/2 nematic liquid crystal is studied. A
feature of twisted nematics is that hydrodynamic flows in Williams domains, together with the tangential component
of velocity, also have an axial component, the direction of which is opposite in neighboring domains. Dislocations can
move both perpendicular (glide) to Williams domains, and along (climb) them. It was found that when dislocations
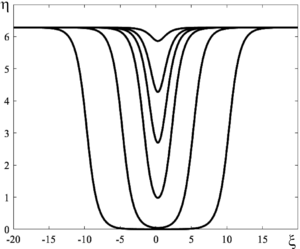
collide with opposite topological charges S = ±1 at given voltage, their speed increases. It has been shown that
dynamics and interaction of dislocations with topological charges S = ±1 are qualitatively well described by the
perturbed sine-Gordon equation.
Keywordsnematic liquid crystal,
domain structure,
dislocation,
kink,
sine-Gordon equation
References
- Cross M.C., Hohenberg P.C. Pattern formation outside of equilibrium // Reviews of Modern Physics. 1993. V. 65. P. 851–1112.
DOI: 10.1103/RevModPhys.65.851
- Pikin S.A. Strukturnye prevrashcheniya v zhidkih kristallah. M.: Nauka, 1981. 336 s. (In Russian)
- De Gennes P.G. and Prost J. The Physics of Liquid Crystals. Oxford: Clarendon, 1994. 596 p.
- Buka A. and Kramer L. (editors). Pattern Formation in Liquid Crystals. N.Y.: Springer-Verlag, 1996. 339 p.
- Kai S., Chizumi N., and Kohno M. Pattern formation, defect motions and onset of defect chaos in the electrohydrodynamic
- Rasenat S., Steinberg V., and Rehberg I. Experimental studies of defect dynamics and interaction in electrohydrodynamic
convection // Physical Review A. 1990. V. 42, No. 10. Pp. 5998–6008.
DOI: 10.1103/PhysRevA.42.5998
- Bodenschatz E., Weber A., and Kramer L. Interaction and dynamics of defects in convective roll patterns of anisotropic
fluids // Journal of Statistical Physics 1991. V. 64, No. 5. Pp. 1007–1015.
DOI: 10.1007/BF01048810
- Joets A. and Ribotta R. Localized bifurcations and defect instabilities in the convection of a nematic liquid crystal //
Journal of Statistical Physics 1991. V. 64, No. 5/6. Pp. 981–1005.
DOI: 10.1007/BF01048809
- Lowe M. and Gollub J.P. Solitons and the commensurate-incommensurate transition in a convecting nematic fluid // Physical Review A. 1985. V. 31, No. 6. Pp. 3893–3897.
DOI: 10.1103/PhysRevA.31.3893
- Lam L. and Prost J. (editors). Solitons in Liquid Crystals. N.Y.: Springer Science & Business Media, 1992. 335 p.
- Frenkel’ YA.I., Kontorova T.A. K teorii plasticheskoj deformacii // ZHurnal Eksperimental’noj i Teoreticheskoj Fiziki. 1938. CH. I. T. 8., Vyp. 1. S. 89–95. (In Russian)
- Braun O.M., Kivshar’ YU.S. Model’ Frenkelya-Kontorovoj. Koncepcii, metody, prilozheniya. M.: Fizmatlit, 2008. 536 s. (In Russian)
- Kivshar Yu.S., Malomed B.A. Dynamics of solitons in nearly integrable systems // Rev. Mod. Phys. 1989. V. 61, No. 4. Pp. 763–915.
DOI: 10.1103/RevModPhys.61.763
- Malomed B.A. Inelastic interactions of solitons in nearly integrable systems. II // Physica D: Nonlinear Phenomena. 1985. V. 15, No 3. Pp. 385–401.
DOI: 10.1016/S0167-2789(85)80006-3
- Ustinov A.V., Cirillo M., Malomed B.A. Fluxon dynamics in one-dimensional Josephson-junction arrays // Physical Review B. 1993. V. 47, No. 13. Pp. 8357–8360.
DOI: 10.1103/PhysRevB.47.8357
- Van der Zant H.S.J., Orlando T.P., Watanabe S., Strogats S.H. Kink propagation in a highly discrete system: observation of phase
locking to linear waves // Physical Review Letters. 1995. V. 74. Pp. 174–177.
DOI: 10.1103/PhysRevLett.74.174
- Cowley R.A., Axe J.D., Iizumi M. Neutron scattering from the ferroelectric fluctuations and domain walls of lead germanate // Physical Review Letters. 1976. V. 36. Pp. 806–809.
DOI: 10.1103/PhysRevLett.36.806
- A.R. Bishop, W.F. Lewis. A theory of intrinsic coercivity in narrow magnetic domain wall materials // Journal of Physics C: Solid State Physics. 1979. V. 12, No. 18. Pp. 3811–3825.
DOI: 10.1088/0022-3719/12/18/027
- Lyuksyutov I.F., Naumovec A.G., Pokrovskij V.L. Dvumernye kristally. Kiev: Naukova Dumka, 1988. 218 s. (In Russian)
- De la Figuera J., Pohl K., de la Fuente R.O., Schmid A.K., Bartelt N.C., Carter C.B., and Hwang R.Q. Direct observation of misfit dislocation glide on surfaces //
Physical Review Letters. 2001. V. 86, No. 17. Pp. 3819–3822.
DOI: 10.1103/PhysRevLett.86.3819
- Ekomasov E.G., Gumerov A.M., Kudryavcev R.V. O Vozmozhnosti nablyudeniya rezonansnogo vzaimodejstviya kinkov uravneniya sinus-Gordona s lokalizovannymi volnami v real’nyh
fizicheskih sistemah // Pis’ma v ZHurnal Eksperimental’noj i Teoreticheskoj Fiziki. 2015. T. 101, Vyp. 12. S. 935–939. (In Russian)
eLIBRARY ID: 23864373
- CHuvyrov A.N., Skaldin O.A., Delev V.A., Lebedev YU.A., Batyrshin E.S. Struktura i dinamika dislokacij Frenkelya-Kontorovoj
pri elektrokonvekcii v zhidkih kristallah // ZHurnal Eksperimental’noj i Teoreticheskoj Fiziki. 2006. T. 130, № 6. S. 1072–1081. (In Russian)
eLIBRARY ID: 9430706
- Delev V.A., Skaldin O.A., Batyrshin E.S., Nazarov V.N., Ekomasov E.G. Kink-antikink vzaimodejstvie v linejnom defekte
elektrokonvektivnoj struktury nematika // Pis’ma v ZHurnal Eksperimental’noj i Teoreticheskoj Fiziki. 2019. T. 109, Vyp. 2. S. 84–88. (In Russian)
DOI: 10.1134/S0370274X19020036
- Delev V.A., Nazarov V.N., Skaldin O.A., Batyrshin E.S., Ekomasov E.G. Slozhnaya dinamika kaskada kink-antikinkovyh vzaimodejstvij v linejnom defekte elektrokonvektivnoj struktury
nematika // Pis’ma v ZHurnal Eksperimental’noj i Teoreticheskoj Fiziki. 2019. T. 110, 607–613. (In Russian)
DOI: 10.1134/S0370274X19210070
- Dodd R., Ejlbek Dzh., Gibbon Dzh., Morris H. Solitony i nelinejnye volnovye uravneniya. M.: Mir, 1988. 694 s. (In Russian)